CBSE Guess > Papers > Question Papers > Class XII > 2005 > Maths > Compartment Delhi Set-I

MATHEMATICS 2005 (Set I—Compartment Delhi)

Q. 1. If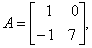

Q. 2. Using properties of determinates prove that

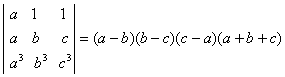
 Q. 3. A machine operates of all of its three components function. The probability that the first component fails during the year is 0.14, the second component fails is 0.10 and the third component fails is 0.05. What is the probability that the machine will fail during the year?

Q. 4. A coin is biased so that the head is 3 times as likely to occur as a tail. If the coin is tossed twice, find the probability for the number of tails.

Or

The probability that a person will get an electric contract is 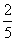and the probability that he well not get plumbing contract is 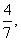if the probability of getting at least one contract 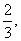is what is the probability that he will get both?

Q. 5. Evaluate: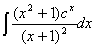

 Q. 6. Evaluate:

Q. 7. Solve the following initial value problem:

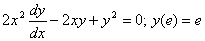
 Q. 8. Solve the following differential equation: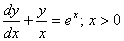

 Q. 9. Examine the validity of the following argument:

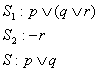
Or

Construct a combinatorial circuit for the following Boolean expression:

• 

• 

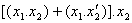
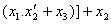
Q. 10. Evaluate: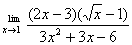

 Q. 11. If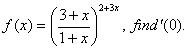

Q. 12. If .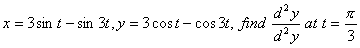

Q. 13. Find the intervals in which the function 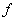given by 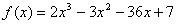is (a) strictly increasing, (b) strictly decreasing.

Q. 14. Evaluate: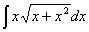

Q. 15. Prove that if is an odd function, them

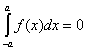
Or

Use it to evaluate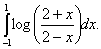

Q. 16. If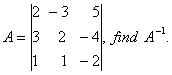

Use it solve the following system of equations:

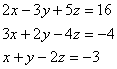
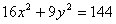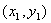Q. 17. Find the equations of the tangent and normal to the curve at where 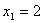and Also, find the points of intersection where both tangent and normal cut the x-axis.

Or

Prove that the volume of the largest cone that can be inscribed in a sphere of radius R is 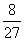of the volume of the sphere.

Q. 18. Using integration, find the area of the smaller region bounded by the ellipse 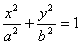and the straight line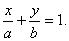

SECTION - B

Q. 19. If 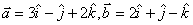and 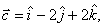then show that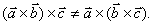

Or

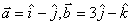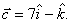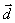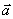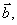Let and find a vector which is perpendicular to both and and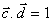

Q. 20. Using vectors, prove that the diagonals of a rhombus are perpendicular bisectors of reach other.

Q. 21. A ball projected vertically upwards takes second to reach a height 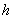metres. If seconds is the time taken by the ball to reach from this point to the ground, prove that 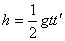and that the maximum height reached is

Q. 22. The velocity v of a particle moving along a straight line when at a distance of x from the origin, is given by 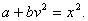show that the acceleration of the particle is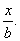

Q. 23. Find the equation of the sphere passing through the origin and making intercepts 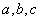on coordinate axes, respectively.

Or

Find the vector and Cartesian forms of the equation of the plane containing two lines

and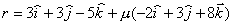

Q. 24. Two forces of magnitudes P+ Q and P-Q make an angle 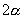with one another and their resultant makes an angle 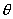with the bisector of the angle between them show that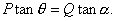

Q.25. ABCD is a square. Along the sides AB, CB, DA forces act equal to 6, 5, 8 and 12 N respectively. Find the algebraic sum of their moments about O, the centre of the square, if the side of the square is 4 m.

Q. 26. Find the Cartesian and vector equations of the planes passing through the intersection of the panes 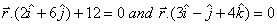which are at unit distance from the origin.

SECTION - C

Q. 19. Two cards are drawn successively with replacement from a well-shuffled pack of 52 cards. Find the mean and variance for the number of aces.

Or

There are 5% defective bulbs in a large bulk of bulbs. What is the probability that a sample of 10 bulbs will includes not more than 1 defective bulb? (Use e- 0.5 =0.6065)

Q. 20. A car manufacturing company has two plants A and B. plant A manufactures 60% of cars and points B manufactures 40% of cars. At plant A, 80% of the cars are rated of standard quality and at plant B, 90% of the cars are rated of standard quality. A car is picked up at random and is found to be of standard quality. Find the probability that it had come from plant B.

Q. 21. Solve the following the linear programming problem graphically:

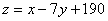
subject to the constraints

Q. 22. A factory owner purchases two types of machines, A and B, for his factory. The requirements and limitations for the machines are as follows:

He has an area of 9000 sq. m available and 72 skilled men who can operate the machines. From a L.P.P. to determine how many machines of each type should he buy to maximize the daily output.

Q. 23. A and B are partners sharing profits and losses in the ratio respectively. They admit C as a new partner, the new profit sharing ratio being 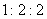between A, B and C, respectively. C pays Rs. 12,000 as premium for good will. How will it be shared between A and B?

Q. 24. A machine, being used by a company, is estimated to have a life of 15 years. At that time, the new machine would cost Rs. 1,48,800 and the scrap of the old machine would yield Rs. 9,200 only. A sinking fund is created for replacing the machine at the end of its life, what sum should be invested by the company at the end of the each year to accumulate at 6% per annum? [Use (1.06) 15 =2.396]

Q. 25. Suppose a manufacturer can sell x items per week at a price 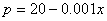rupees each when it costs 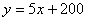rupees to produce items. Determine the number of items he should produce per week for maximum profit.

Or

A firm suffers loss of Rs. 144 when there is no sales. The marginal revenue is given by 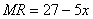and marginal cost by .

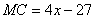Q. 26. A bill for Rs. 10,100 is drawn on May 10. It is discounted on JULY 11 at 5% per annum. If the banker's gain in the transaction is Rs. 1, find the nominal date f maturity of the bill.